 Below is the transcript of my tutorial video on MCAT Physics Video 8 – Projectile Motion in Translational Motion
Below is the transcript of my tutorial video on MCAT Physics Video 8 – Projectile Motion in Translational Motion
(Click Here to watch on YouTube)
[Start of Transcript]
Leah here from leah4ci.com/MCAT and in this video I’ll show you how to tackle projectile motion questions as they show up on your MCAT. You can find my entire series on Translational Motion by visiting my website https://leah4sci.com/MCATPhysics.
On the MCAT or Physics in general, a Projectile tends to refer to a rocket or catapult. Something that is launched. A Projectile is something that has an initial velocity somewhere between the X and Y direction. So we’ll call that Velocity Initial and to figure out components such as height, time, distance and so son, you have to break it up into its vector components to find Vx solving for the X-direction problems and Vy to solve for Y-direction problems.
Let’s summarize before going into a problem by describing what I like to think of as the Looney Tunes Effect. If something is shut off at an initial velocity but you’re not given an acceleration, then the only acceleration of the problem is that in the Y-direction because your Velocity initial breaks down into a standard X and Y velocity but Gravity is at downward acceleration that is always constant.
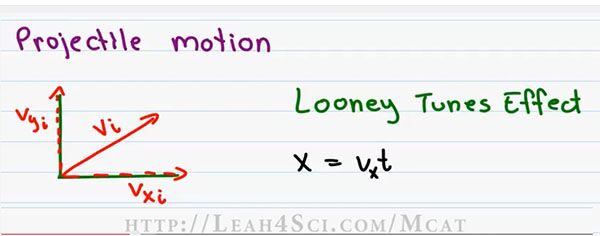
To find the distance that a projectile traveled, you would use an X equation that does not account for acceleration and that is X is equal to Vt. The V being the X. In order to find that Vx, you simply break the V initial into its X component, but to find the time, you have to refer back to the Y-equations.
You have your initial Y velocity going up but the Y acceleration gravity going down so as the projectile’s going up up up, gravity slows it down to the point where it gets to that height where the velocity slows down to a zero. That means it’s at the highest point that it’ll be and then changes Velocity to fall straight down going faster and faster until it hits the ground.
The X and Y components are completely independent of each other in this respect and this is where the Looney tunes idea comes in. if you watch cartoons as kid, think back to the roadrunner cartoons where the Cayote is chasing the roadrunner and it keeps on running and running. And he keeps running on running right past the edge of the cliff until suddenly he looks down and realizes there’s nothing under him and he crashes straight to the ground.
That kind of what’s happening. Just imagine the projectile takes off and then travels at a consistent Velocity on the X direction until the time runs out and it’s like cayote looks down and just crashes right there.
So to find the distance, we need to find the time where the Cayote essentially realizes he’s in mid-air that’s where time’s going to end and we find that from the Y components.
Now one more thing to keep in mind is on the MCAT, you’re not going to have a question like this that has you solve multiple simultaneous equations. But, you may find yourself having to solve one component of Projectile Motion so it’s important for you to know what to do every step of the way in case that is the step you’re tested on.
Let’s apply this concept to this question.
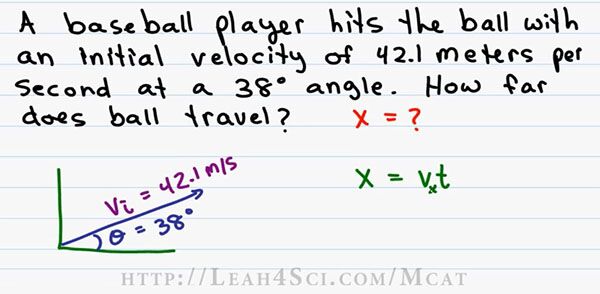
A baseball player hit the ball with an initial velocity of 42.1 meters per second at a 38 degree angle. How far does the ball travel?
The first we want to do is get an idea of what’s going on and since we have an initial angle and initial Velocity given, let’s draw it out. The angle theta is given as 38 degrees with an initial velocity of 42.1 meters per second. We’re asked how far does the ball travel which means we’re asked to solve for X.
Since there is no acceleration in the X direction, we’ll use the acceleration independent equation, something I explain in video 9 in this series and that is X is equal to Vt or specifically the Vxt. Let’s start by solving for Vx.

We know that we’ll need Vy so let’s solve for both simultaneously. We’ll use the trick of Vx is equal to Vcosx spelled with x theta and Vy is equal to Vsyn spelled with the Y times feda.
If you’re not comfortable with this shortcut go back to my MCAT Trigonometry Video which you can find on my website at https://leah4sci.com/MCATMath.
The next thing to do is find the values of syn 38 and cosx 38. On my MCAT Math Cheat Sheet also on that page, I give you the angles to memorize for thirty, forty five, sixty, ninety. But know that we have 38 and you don’t want to memorize all the different angles instead I want you to figure out how you can find it.
Thirty eight (38) is somewhere between the angles of 30 and 45. So if we can find the cosx values and syn values for 30 and 35, we’ll take the middle number and use that as your estimation.
Sin of 30 is 0.5, sin 45 is 0.7, Cos 30 is 0.9, Cos 45 is also 0.7. The halfway between 0.5 and 0.7, gives us Sin is equal to 0.6, the halfway between 0.9 and 0.7 gives us a cos of 0.8. Now let’s do the Math. V cos theta is 42 times 0.8. If this is hard to do in your head, let’s try the decimal trick, we’ll move one to the right and one to the left, now we have 8 times 4 which is 32.
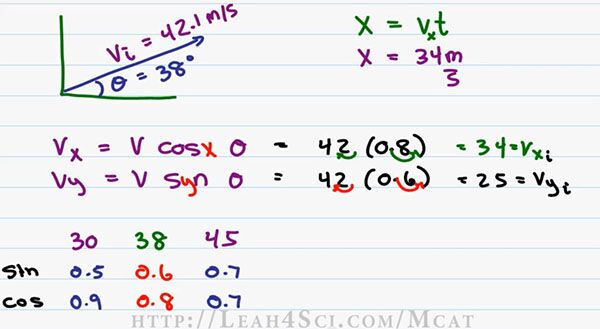
If you want a round a little closer, you can also do eight times point two (8x.02). Eight times two is sixteen which is close to twenty so eight times point two is about two. Let’s make this thirty four and this is Vx.
For Vy, we have 42 times 0.6. We’ll do the trick again, decimal to the right, decimal to the left. Six times four is twenty four. For six times point two, we have six times to which is twelve, so six times point two is one point two. Let’s raise this by one giving as 25 as our Vy initial, that’s the X initial.
Going back to our initial equation, we have that x is equal to Vx of 34 meters per second but we still need the time. In order to find the time, we have to use the Y components to see how long it takes this Projectile to get to the top. But remember, that’s only half of the total time. You have the time it takes for the ball to get to the maximum height and then the same time for the ball to get from the maximum height down to the starting height.
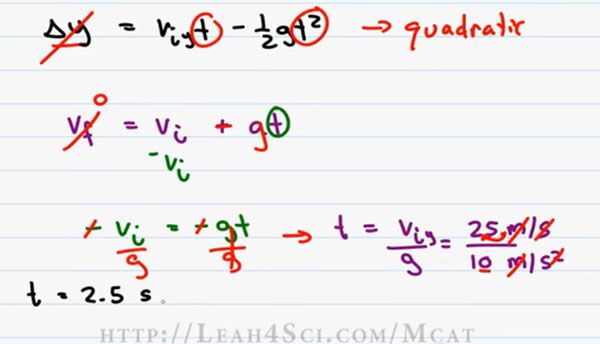
It gets a little more confusing when you wanna solve something like time. From your Physics class, you may want to use your delta Y is equal V initial yt minus one over two gt squared. This comes from your V initial t plus a half eighty squared but gravity is downward so we changed a to g and plus to minus.
We have two problems with this equation. One, we don’t know about delta y. Yes, we can solve for delta y but we were never asked for it so we don’t care about it. Even if we did solve for delta y from another equation, having velocity times t and here t squared, that will give us a quadratic and that is something you want to avoid at all cost on the MCAT.
So looking back on your list of equations, again something I’ll talk about in the next video we want to come up with the y independent equation. That one that only has Velocity, Acceleration and Time. And that equation is V final is equal to V initial plus at. Given that we’re dealing with the y-direction, we have negative because acceleration is down and g because we’re dealing with acceleration of gravity.
Since we’re solving from the moment the baseball was hit until the time it takes to reach to the top. V final is equal to zero because the Velocity stops before it starts to plummet. So let’s go ahead and isolate t. We’ll subtract the initial from both sides giving us negative V initial is equal to negative gt. We’ll cancel out the negatives giving up positive values to work with and then we’ll divide both sides by g giving us the new equation t is equal to V initial y over g (t=Viy/g).
We got the V initial y as 25 meters per second and gravity on the MCAT have estimates to 10 meters per second squared. Cancel the units first, meters cancel, seconds cancels the square, we have one over one second which is second. Twenty five divided by ten has that factor of 10 trick. We have one zero that means we move the decimal back one space which gives us time is equal to 2.5 seconds. But remember this is only half the time or the time it takes to get to the top.
If you’re not comfortable with this, let’s take a look at the path of the baseball. The baseball is hit and then it goes up and up and up until it reaches V top which is equal to 0 and this happens to be your Ymax and then gravity takes over giving you an increasing Velocity going down until we hit the bottom.
The velocity going up is the inverse of the velocity going down. Because going up go slower, slower, slower, going down we go faster, faster, faster. And so we call this t1, that’s time to get to the top. T2 will be the time to get to the bottom but they’re equal so all we have to do is take our time and multiply by 2 to get the total since we’re starting and ending at approximately the same height so we have two point five times 2 which is a total of five seconds.
Now that we have the time, we can go back to that initial equation where we have x is equal to V initial xt (Vit) and that’s 34 meters per second times 5 seconds. The seconds cancel out leaving us with meters as the answer which is good because we’re looking for a distance.
Thirty four times five may not be as easy to do in your head but thirty times five is a hundred and fifty coz three times five is fifteen, four times five is twenty, adding them up we get a 170 meters.
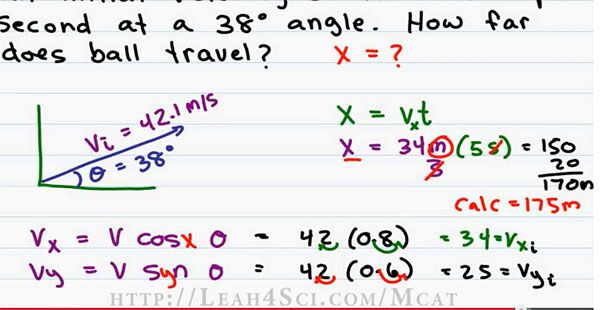
Now I realized we did a lot of rounding and estimation on this problem but on the MCAT that’s fine because an answer that s close enough is good enough but just to show you how close we are, I punched these numbers into the calculator and got a distance of 175 meters which as you can see is very very close to our answer.
Be sure to check out the next video where I take you through the individual Kinematic equations showing you how they relate to different forms you may have learned and then I’ll show you how to decide which equation to use for any given problem so that you don’t waste time trying to go through all of them.
You can find this video alongwith my entire series on Translational Motion on my website at https://leah4sci.com/MCATPhysics.
Are you stuck on a specific MCAT topic? I offer Private Online Tutoring where I focus on your needs to strengthen your individual weaknesses. Tutoring details can be found using the link below or by visiting my website leah4sci.com/MCATTutor.
Are you overwhelmed by the sheer volume of information required for the MCAT? Are you worried that lack of a proper study plan and low MCAT score will prevent you from getting into Medical School? My new eBook The MCAT Exam Strategy is 6-Week Guide to Crushing the MCAT will help you formulate a concrete study plan by helping you figure out where you stand now, identify your goals and figure out what it takes to reach them and it’s yours FREE when you sign up for my email newsletter at McatExamStrategy.com. By signing up for my email newsletter, you’ll also be the first to know when I have new videos, MCAT Study Guide Cheat Sheets, Tips and so much more! The link again McatExamStrategy.com.
[End Transcript]
Watch The Video Here: Translational Motion Projectile Motion